When Is a Matrix Orthogonally Diagonalizable

By Ra_Michaela973 11 Apr, 2022 Post a Comment

Online tool orthorgnol diagnolize a real symmetric matrix with step by step explanationsStart by entering your matrix row number and column number in the formula pane below. A non-diagonal 2 2 matrix for which there exists an orthonormal eigenbasis you do not have to nd the eigenbasis only the matrix 3.

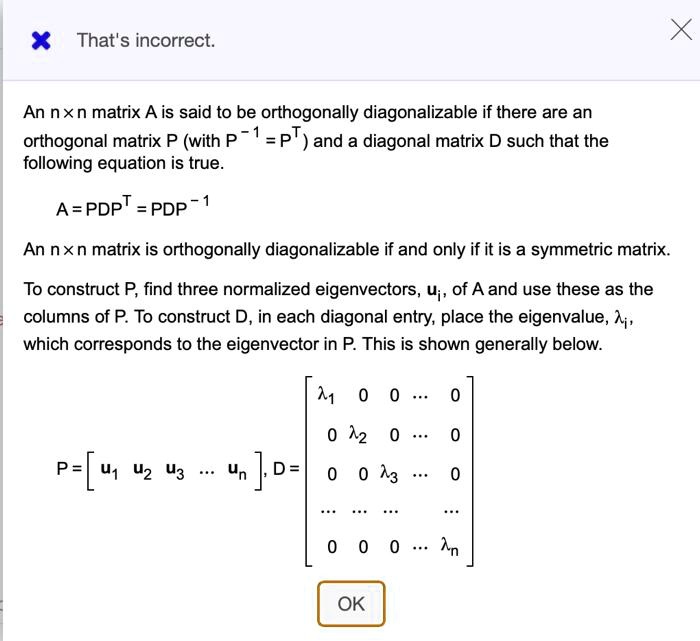
Solved X That S Incorrect An Nxn Matrix A Is Said To Be Orthogonally Diagonalizable If There Are An Orthogonal Matrix P With P Pt And A Diagonal Matrix D Such That The Following

Orthogonalization is used quite extensively in certain statistical analyses.

. Orthogonal matrix is a square matrix with orthonormal columns. If Ais symmetric then there is a matrix Ssuch that STASis diagonal. A matrix A is orthogonally diagonalizable if there is an orthogonal matrix P that diagonalizes it.

A n x n matrix M is said to be orthogonallyunitarily diagonalizable if there exists an orthogonal or unitary n x n matrix U such that for a diagonal n x n matrix D. D a 1 1 0. A matrix is diagonalizable by a unitary matrix if and only if it is a normal matrix.

I know that orthogonally diagonalizable means that you can find an orthogonal matrix Q and a Diagonal matrix D so QTAQD AQDQT. Spectral theorem A2R n is orthogonally diagonalizable if and only if it is symmetric. Orthogonally Diagonalizable Matrices A matrix A is orthogonally diagonalizable if there is an orthogonal ma- trix P that diagonalizes it.

If an ntimes n matrix A is diagonalizable then there exists an invertible matrix P such that P-1APbeginbmatrix. Thus an orthogonally diagonalizable matrix is a special kind of diagonalizable matrix. Orthogonally diagonalizing Symmetric Matrices.

Free Matrix Diagonalization calculator - diagonalize matrices step-by-step This website uses cookies to ensure you get the best experience. However the zero matrix is not invertible as its determinant is zero. 0 0 a 2 2 0.

A n n D 1 1 a 1 1 0. It is gotten from A by exchanging the ith row with the ith column or by reﬂecting across. Show that the matrix.

Show that the matrix A -. 82 Orthogonal Diagonalization Recall Theorem 553 that an nn matrix A is diagonalizable if and only if it has n linearly independent eigenvectors. Let us give a more theoretical explanation.

That is a matrix is orthogonally diagonalizable if and only if it is symmetric. Definition An matrix is called 88 E orthogonally diagonalizable if there is an orthogonal matrix and a diagonal matrix for which Y H EœYHY ÐœYHY ÑÞ X Thus an orthogonally diagonalizable matrix is a special kind of diagonalizable matrix. If matrix P is an orthogonal matrix then matrix A is said to be orthogonally diagonalizable and therefore the equation can be rewritten.

Chapter7 Diagonalization and Quadratic Forms True-False Exercises a The matrix 0 1 0 0 is orthogonal. By using this website you agree to. An n n matrix is called orthogonally diagonalizable if there is an orthogonal matrix U and a diagonal matrix D for which A U D U 1 U D U.

0 0 1 a 2 2 0. An orthogonally diagonalizable matrix is necessarily symmetric. If latexBPDPTlatex where latexPTP-1latex and latexDlatex is a diagonal matrix then latexBlatex is a symmetric matrix.

B The matrix 1 2 2 1 is orthogonal. By Proposition 231 is an eigenvalue of Aprecisely when det I A 0. The zero matrix is a diagonal matrix and thus it is diagonalizable.

Not only can we factor APDP1 but we can find an matrix that UP. An important consequence of this is that a symmetric n nmatrix has counting multiplicities exactly nreal eigenvalues EXAMPLE. Every orthogonal matrix is orthogonally diagonalizable.

For each item nd an explicit example or explain why none exists. P 1 P T P -1PT P 1 P T. Moreover the matrix P with these eigenvectors as columns is a diagonalizing matrix for A that is P1AP is diagonal.

The dimension of an eigenspace of a symmetric matrix equals the multiplicity of the corresponding eigenvalue. D A square matrix whose columns form an orthogonal set is orthogonal. Every symmetric matrix is orthogonally diagonalizable.

Not only can we factor A P D P 1 but we can find an matrix that U P that works. C An m x n matrix A is orthogonal if A T AI. UDU 1.

An orthonormal eigenbasis for an arbitrary 3 3 diagonal matrix. Fix a matrix A6 kI n for any scalar k. Although some matrices can never be diagonalized.

So lets nd the eigenvalues and eigenspaces for matrix A. An nn matrix is called orthogonally diagonalizable if there is an orthogonal matrix U and a diagonal matrix D for which AUDU1UDU. 4 21 2 is orthogonally diagonalizable.

A real square matrix A is orthogonally diagonalizable if there exist an orthogonal matrix U and a diagonal matrix D such that A UDUmathsfT. 1 a n n Dbegin pmatrix a_ 110dots0 0a_ 22vdots vdotsddots 0dotsa_ nn end. Note I A 2 4 6 3 8.

Orthogonally diagonalize A 2 4 1 1 1 1 1 1 1 1 1 3 5 This is symmetric so can be orthogonally diagonalized by the spectral theorem. If Ahas an orthonormal eigenbasis then every eigenbasis is orthonormal. If A aij is a not neces- sarily square matrix the transpose of A denoted AT is the matrix with ij entry a ji.

Thus an orthogonally diagonalizable matrix is a special kind of diagonalizable matrix. I am aware of the Real Spectral Theorem which states that A real mxn-matrix A is orthogonally diagonalizable if and only if A. Symmetric matrices have orthogonal eigenvectors.

By Proposition 232 matrix Ais diagonalizable if and only if there is a basis of R3 consisting of eigenvectors of A. Is orthogonally diagonalizable second part of the assumption and that. A matrix is diagonalizable by a unitary matrix if and only if it is a normal matrix.

A matrix N is said to be normal if N N. Consider the linear transformation Rn n f. If Pis any 5 9 matrix then PPT has an orthonormal eigenbasis.

Given two diagonalizable matrices they commute if and only if they can be diagonalized simultaneously that is if they share the same orthonormal basis of eigenvectors.

Orthogonal Diagonalization 1 Youtube